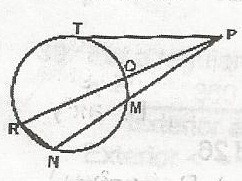
PMN and PQR are two secants of the circle MQTRN... - JAMB Mathematics 1991 Question
A
7.3, 5.9
B
7.7, 12.5
C
12.5, 7.7
D
5.9, 7.3
Ask EduPadi AI...
Correct Option: C
(\frac{PQ}{PN} = \frac{PM}{PR} = \frac{QM}{NR})
(\frac{4.8}{12} = \frac{5}{PR})
PR = (\frac{5 \times 12}{4.8} = \frac{50}{4})
= 12.5
(\frac{PQ}{PN} = \frac{PM}{PT} = \frac{TM}{NT})
(\frac{PT}{12} = \frac{5}{PR})
PT2 = 60
PT = (\sqrt{60})
= 7.746
= 7.7
Please share this, thanks:
#JAMB #JAMB
Add your answer
No responses